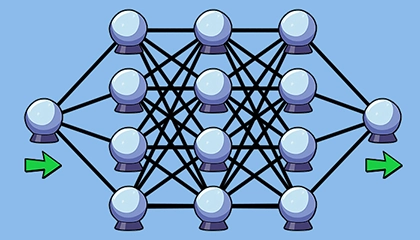
How Does ChatGPT Work?

The Life of Elon Musk

Viruses: Genes Gone Rogue

16 Giant Leaps in Animal Evolution

How Does DNA Work?
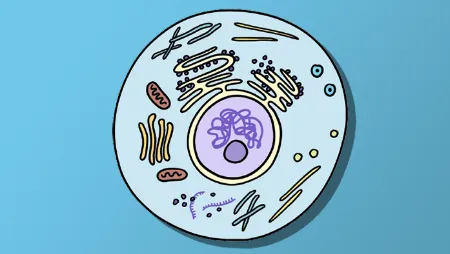
Where Do Cells Come From?
How Does Gene Therapy Work?

The State of Climate Change

How Does Evolution Work?

Helium Could Save Our Planet

How Does Conditioning Work?